P. He, X. Zhang1,2,3, J. Zhang1, Z. Ji1, Y. Zhao1, H. Xu4, M. He1, Z. Zhang1, M. Zou1, H. Chen1, Y. Qiu1, J. Yang1 and L. Zhang1*

School of Life Science, Zhengzhou University, Zhengzhou, 450001, China
1Institute of Medical and Pharmaceutical Science, Zhengzhou University, Zhengzhou, 450001, China
2School of Mechanics and Engineering Science, Zhengzhou University, Zhengzhou, 450001, China
3National Center for International Joint Research of Micro-Nano Molding Technology, Zhengzhou University, Zhengzhou, 450001, China
4First Affiliated Hospital of Zhengzhou University, Zhengzhou University, Zhengzhou, 450001, China

*Corresponding Author:
L. Zhang
Institute of Medical and Pharmaceutical Science, Zhengzhou University, Zhengzhou, 450001, China
E-mail: docgpy@126.com
Date of Submission 12 June 2016
Date of Revision 08 September 2016
Date of Acceptance 30 October 2016
Indian J Pharm Sci 2016;78(6):708-714  

This is an open access article distributed under the terms of the Creative Commons Attribution-NonCommercial-ShareAlike 3.0 License, which allows others to remix, tweak, and build upon the work non-commercially, as long as the author is credited and the new creations are licensed under the identical terms

DOI: 10.4172/pharmaceutical-sciences.1000174

Abstract

In the drug delivery systems using external ultrasonic stimulation to manipulate the drug release from carriers, the effect of the ultrasonic stimulation on drug carriers should be investigated. Alginate beads were fabricated using a conventional extrusion method, and their shear moduli were calculated by a compression test and the hertz law. The changes in the shear moduli of the alginate beads resulted in changes in their physical properties. Alginate is a thermally sensitive material. Ultrasonic stimulation increased the temperature of the solution. When sonicated at high temperatures, the alginate beads shrank and their shear moduli increased exponentially. In the absence of the ultrasonic thermal effect, no change in the physical properties of the alginate beads was evident. Therefore, the ultrasonic thermal effect influenced the physical properties of the alginate beads. The stream and cavitation effects produced by the ultrasonic stimulation did not affect the properties of the alginate beads. it can be attributed to the fact that alginate beads have a high shear moduli and that the ultrasonic cavitation cannot damage the surface of alginate beads.

Keywords

Ultrasonic stimulation, alginate beads, shear module, thermal effect

Ultrasonic stimulation has been used in drug delivery systems as an external stimulation to manipulate the drug release from different carriers such as beads, capsules, liposomes, and polymeric micelles [1]. Each type of carrier has its own release behavior, which can be controlled by varying the power, frequency, and duration of the ultrasonic stimulus. The primary reasons of this change in the release behavior are the cavitations, thermal effect and acoustic streaming produced by the propagation of ultrasonic waves in solutions [2].

Under an ultrasonic stimulation, the cavities implode and generate extremely high energy shock waves. This phenomenon can be used to scissor polymer molecules in solutions [3] and also to degrade the polymer matrix surfaces. Kost et al. reported that an increase up to five-fold is observed in the degradation rate of polyanhydrides, polyglycolides and polylactides when they are exposed to an ultrasonic stimulus of 5 w/cm2. The main chain rupture of the polymers was thought to be induced by the shock waves created during the cavitation. However, the ethylene-vinyl acetate (EVA) copolymer matrices exposed to an ultrasound stimulus of 2.5 w/cm2 do not show any degradation [4]. Thermal effect is concomitantly produced by the implosion of cavities and energy conversion during the propagation of ultrasonic waves. It increases the temperature of the aqueous solution and affects the materials in the solution. The thermal effect is especially evident on thermo-responsive materials. The radiation pressure force causes acoustic streaming, which tends to push the target material along the direction of wave propagation. Passive cell membrane permeability has been observed in vitro in the systems where it is possible to produce ultrasonic streaming.

Alginate is a biocompatible, degradable and non-toxic biomaterial [5,6]. It has been widely used as a drug carrier and cell culture environment material [6]. Ultrasonic stimulation of alginate gel improves the drug release or changes the absorption of ions [7]. Alginate gel is a thermo-sensitive material [8]. Physical changes of alginate can be shown in physical properties [9]. Ultrasonic stimulation induces three main effects: acoustic streaming, acoustic thermal effect and cavitation [10]. The response of alginate beads to an ultrasonic stimulus can be detected by investigating their physical properties [11].

Materials and Methods

Fabrication of alginate beads

A conventional extrusion method was used for the fabrication of alginate beads. First, alginate powder (2 g, A0682, Sigma Aldrich, USA) was dissolved in distilled water (100 ml) to form a 2% alginate solution. A peristaltic pump (Ismatec ISM834C, Switzerland) was used to exert an extrusive force on the alginate solution to maintain a flow rate of 1 ml/min at room temperature. Micro-droplets consisting of alginate solution were formed at the tip of a needle (24 gauge, Fisher Scientific). These micro-droplets were then made to fall into a tanker filled with 50 ml of 1.7% calcium chloride solution. The tip was placed at a distance of 10 cm above the solution surface to ensure that the micro-droplets were spherical. Polymerization occurred once the micro-droplets contacted the calcium chloride solution, and the process lasted for 20 min. The micro-sized beads were then finally stored in the 1.7% calcium chloride solution for 1 d before being used in the subsequent experiments.

Measurement of shear modulus of alginate bead

A compression method was used to measure the bead deformation and the compression forces exerted on beads. The beads were compressed between two rigid parallel plates and the varying distance between the two plates was recorded. Although different theoretical models have been used to experimentally evaluate the compression depending upon the geometry of samples [10], we used the Hertz law [11] (Eqn. 1 shown below), to calculate the shear moduli of the solid spherical beads obeying Hooke's law. The compression force variation for a spherical bead positioned between the two rigid plates is expressed as Eqn. 1: F(t)=8μr20(δ(t))1.5/3(1-v), where μ and ν are the shear modulus and Poisson’s ratio of the bead material, respectively, and r20 is the original radius of the bead. Since the Poisson’s ratio of alginate beads has been found to be 0.5 [12], the force F(t) predicted by the Hertz law therefore becomes Eqn. 2: F(t)=16μr20(δ(t))1.5/3. Thus, we deduced μ for each pair of F(t) and δ(t) values, which were measured experimentally.

Compression test on alginate beads

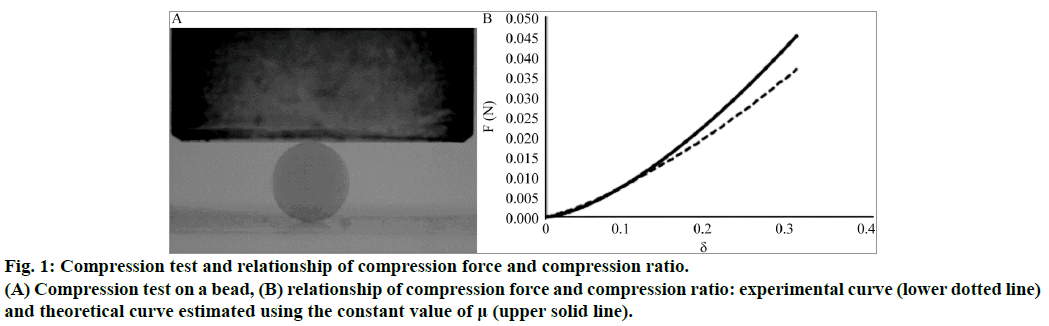
A computer controlled traction/compression device (Synergie 400, MTS Systems, France) was fitted to a 2 N force transducer (accuracy 10-4 N). An alginate bead was placed on the lower plate within a transparent cup filled with the 1.7% calcium solution and its shape was continuously monitored by a CCD camera (JAIM150, Imasys S.A., France). The images were captured with the Scion Image software (Scion Image, Scion Corporation, USA) and were analyzed with Image J 1.42 q (National Institutes of Health, USA). As shown in (Figure 1A), contour analysis was performed to detect the bead edge and the initial height (D0) and width (L0) of the bead. The bead was almost spherical and its volume was computed assuming it to be axisymmetric. The apparent radius (r0) of the bead, which is defined as the radius of a sphere with a volume equivalent to the measured bead volume, was calculated to be 1.12±0.01 mm. The alginate bead was compressed by a piston which moved down at a constant speed of 0.6 mm/ min. This speed was low enough to eliminate the effect of inertia and large enough to avoid potential osmotic effects. At each time step, the acquisition system automatically recorded the imposed displacement of the piston D(t) and the resultant force exerted on the piston.

ijps-compression-force

Figure 1: Compression test and relationship of compression force and compression ratio.
(A) Compression test on a bead, (B) relationship of compression force and compression ratio: experimental curve (lower dotted line) and theoretical curve estimated using the constant value of μ (upper solid line).

The initial contact point between the piston and the bead corresponded to D (0)=0. It was determined with a precision of ±20 μm. The ratio D (t)/D0 (δ) is the compression ratio. The buoyancy force acting on the piston was subtracted from the measured force to determine the net force F(t) acting on the bead. The variation of F(t) as a function of δ represents the compression curve.

Ultrasonic stimulation setup and measurement

Every six beads were collected to form a batch and were put into a tube with 2.5 ml of the 1.7% calcium chloride solution. The batch was dispersed in the solution and was ultrasonically stimulated by a sonotrode (7 mm diameter) (MS7, Hielscher, Germany) assembled in a 30 kHz ultrasonic generator (UP50H, Hielscher, Germany). To investigate the influence of ultrasonic stimulation on the alginate beads, three parameters of the ultrasonic generator were changed: the pulse mode percentage (fp), ultrasonic power (Ps), and ultrasonic duration (ts). Three sets of experiments were carried out. In each set, one of the parameters was varied by keeping the other two fixed to determine the effect of the varying parameter on the shear modulus of the alginate beads. Each experiment was conducted on six alginate beads. Their shear modulus (μ) was measured by compression using the Hertz model after the stimulation. Their diameters (D) were measured from the captured images.

Effect of thermal stability on the shear modulus of alginate beads

A big container filled with 1 l of the 1.7% calcium chloride solution was used as the cooling tank. For conducting the stimulative experiment, the alginate beads were put in this container. The modulus of the alginate beads kept in a small tube varied under the influence of the ultrasonic stimulation. The cooling equipment absorbed the heat energy produced by the ultrasonic stimulation and maintained the temperature of the solution at room temperature. In these experiments also, we could change the three parameters of the ultrasonic generator mentioned above. Three sets of experiments were carried out. In each experiment, one of the parameters was varied by keeping the other two constant to determine the effect of the varying parameter on the mechanical properties of the alginate beads. In the Hertz model, a batch of every sixth alginate bead was compressed to monitor the mechanical properties of the beads.

Results and Discussion

Measurement of shear modulus of alginate beads, from the compression test on a stable alginate bead, we determined the variation of F(t) as a function of δ(t). The compression force varied non-linearly with the deformation because the contact area between the alginate bead and the plates varied during the compression. For every pair of F(t) and δ(t) values measured experimentally, the value of μ(t) was calculated. The shear modulus was relatively constant for compression ratios in the range of 5-15%, as shown by a good fit between the experimental and theoretical compression curves in Figure 1B. The shear modulus of the alginate bead was found to be around 42.5 kPa.

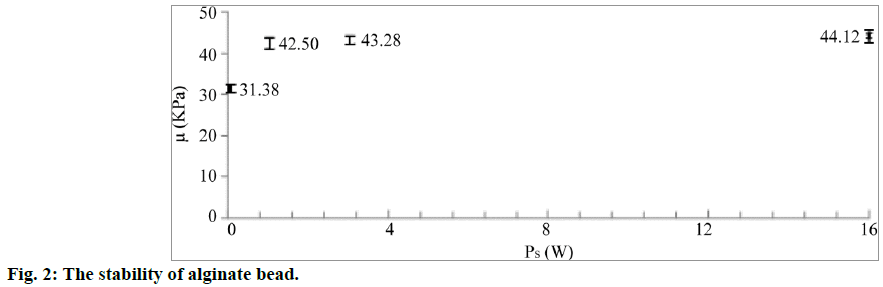
The alginate beads were divided into four batches depending upon the duration for which they were conserved in 50 ml of the 1.7% calcium solution (1 h, 1 d, 3 d and 16 d). Each batch had 50 beads. The shear modulus of the beads was calculated from the results of the compression test. The measurements were repeated on a minimum of six beads for each batch. The average value of the shear modulus was calculated for the compression ratios in the range of 5-15%. The result is shown in Figure 2. The alginate beads conserved for 1 h had a shear modulus of 31.4 kPa. The shear modulus of the alginate beads increased with an increase in the conservation time. After the conservation for 24 h, the shear modulus became quasi-constant. The differences between the values obtained for the three datasets were within the experimental error range.

ijps-alginate-bead

Figure 2: The stability of alginate bead.

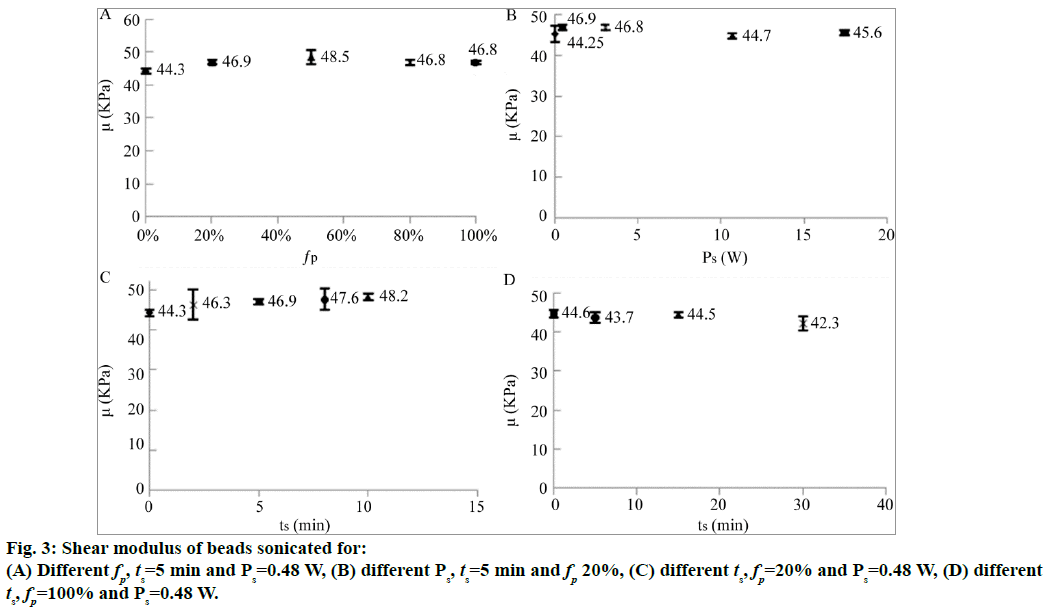
Five batches of the preserved alginate beads were sonicated at Ps=0.48 W for 5 min at different fps. The compression test results revealed that an increase in fp had no influence on the shear modulus of the alginate beads (Figure 3A). The five batches of the preserved alginate beads were then sonicated at 20% fp for 5 min at different sonication powers (Ps). The sonicated beads were compressed and then analyzed using the Hertz model. Their shear moduli are shown in Figure 3B. In this case too, the sonication did not influence the shear moduli significantly.

ijps-Shear-modulus

Figure 3: Shear modulus of beads sonicated for:
(A) Different fp, ts=5 min and Ps=0.48 W, (B) different Ps, ts=5 min and fp 20%, (C) different ts, fp=20% and Ps=0.48 W, (D) different ts, fp=100% and Ps=0.48 W.

The sonication of the five batches of the preserved alginate beads was then carried out by varying the stimulation duration (ts) and keeping the parameters fp and Ps fixed at 20% and 0.48 W, respectively. The shear moduli are shown in Figure 3C. Only a small increase was observed in the shear moduli with an increase in the sonication time. Since, the variations were smaller than the experimental error, nothing can be said about the significance of this result.

Longer sonication times were thus tested with a continuous stimulation to maximize the pulse mode 100% with a power of 0.48 W. The shear moduli of the sonicated beads compressed and analyzed using the Hertz model are shown in Figure 3D. The results shown in Figure 3D were obtained at the same sonication power as that used for obtaining the results in Figure 3C. The results indicate that the sonication time did not affect the mechanical properties of the alginate beads.

The absence of stimulation in our experiments correspond to the condition of Ps=0, fp=0, ts=0, temperature=20.4°. The first set of experiments was carried out at fp=20%, ts=5 min by varying the Ps from 0.48 to 17.46 W. The changes in the physical properties of the beads after the ultrasonic stimulation are given in Table 1. The second set of experiments was carried out at Ps=0.48 W, ts=5 min by varying the fp from 20 to 100%. The changes in the physical properties of the stimulated beads are given in Table 1. The third set of experiments was carried out at Ps=0.48 W, fp=20% by varying the ts from 2 to 5 min. The corresponding results are given in Table 1.

  Ps(W) fp ts(min) Ts(°) D (mm) μ (kPa)
Different PS
1 0 0 0 20.4 2.02 42.86
2 0.48 20% 5 29.1 2.01 46.10
3 3.06 20% 5 34.3 1.98 48.28
4 1.71 20% 5 41.7 1.96 50.89
5 17.46 20% 5 43.4 1.94 51.01
Different FP
1 0 0 0 20.4 2.02 42.86
2 0.48 20% 5 29.1 2.01 46.10
3 0.48 50% 5 47.5 1.94 54.41
4 0.48 80% 5 63.7 1.86 64.15
5 0.48 100% 5 75.7 1.81 80.46
Different TS
1 0 0 0 20.4 2.02 42.86
2 0.48 20% 2 25.4 2.02 44.85
3 0.48 20% 5 29.1 2.01 46.10
4 0.48 20% 8 32.3 1.99 46.58
5 0.48 20% 10 35.0 1.97 48.40

Table 1: Changes of Physical Properties of Beads Stimulated by Different Ps (A), Different Fp(B) And Different Ts (C)

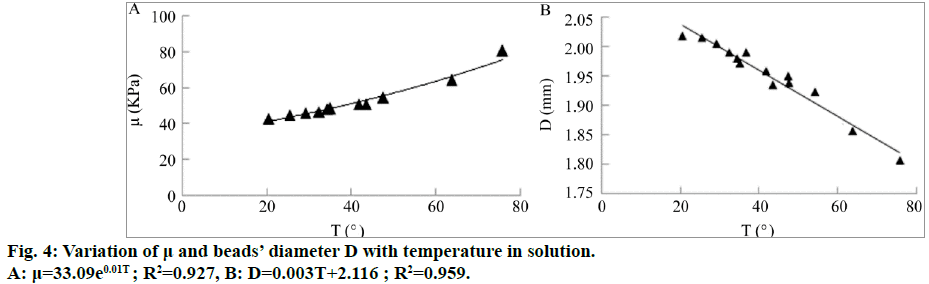
From the three tables, it can be observed that the temperature of the stimulated solution in the tubes increased with an increase in Ps, fp and ts. This can be due to the conversion of the ultrasonic energy into heat energy during the stimulation. An increase in the solution temperature causes alginate gel to shrink (Figure 4A). The shrinking ratio can be up to 10% [13] and has a linear relationship with the temperature [14]. Thus, we can state that the exponential increase in the shear moduli of alginate beads arises because of the shrinkage of the beads (Figure 4B).

ijps-temperature-solution

Figure 4: Variation of μ and beads’ diameter D with temperature in solution.
A: μ=33.09e0.01T; R2=0.927, B: D=0.003T+2.116 ; R2=0.959.

The shear modulus of the alginate beads in our study was found to be around 42.5 kPa and was relatively constant for the compression ratios in the range of 5-15%. Hence, a good fit between the experimental and theoretical compression was obtained. Ouwerx et al. [15] have previously characterized alginate beads made up of 1.5% alginate solution by compression. They obtained a shear modulus of about 53 kPa, which is higher than that obtained by us. The difference may be due to the method used for the compression. Ouwerx et al. conducted the compression on slightly dried beads, which resulted in an increase in the shear moduli of the beads. Leroux et al. [16] conserved the alginate beads fabricated using a 2.0% alginate solution in 0.0018 M (0.02% w/v) calcium solution for 15 h. They obtained a shear modulus of 24 kPa. This value was lower than that obtained by us. This difference is attributed to the difference in the calcium solution concentration (0.02% vs. 0.8% in our case).

Gelation of alginate is required for achieving a stable state and is time consuming process [17]. On the basis of our results, we can conclude that a gelation time of 24 h is sufficient to stabilize the alginate gel, and its stability can be sustained for at least 16 d. According to some previous reports, alginate beads reach their stable state after about 15 h of conversation in a calcium solution. Hence, the gelation time used in this study was optimum.

Ultrasound assisted and pre-ultrasound treatments have been used for enzyme immobilization. It has been shown that the diffusion coefficient (De) increases with an increase in ultrasound power until it reaches the highest value, which is determined by the theory of enzyme immobilization using ultrasound assisted treatment [18]. Considering the ultrasonic stimulation of alginate beads, Ciuti et al. [19] reported that an increase in fp supplies a high ultrasonic power to the sonicated volume. Nevertheless, in our study, we hardly observed any significant variation in the mechanical properties of the beads. This difference could be a result of using the cooling equipment in our experiments.

At room temperature, alginate gel is relatively stable. The results obtained in this study suggest that an increase in the solution temperature results in the shrinking of alginate beads and an increase in their shear moduli. The diameter of the alginate beads decreased with an increase in the temperature. This observation was consistent with that reported by D Serp [20]. As shear modulus is a function of bead diameter, the shear modulus should also show the same behavior. Indeed, D Serp confirmed that the mechanical resistance of alginate beads increases with the increasing temperature.

In conclusion, we investigated the mechanical properties of alginate beads by carrying out a compression test. The analysis was carried out using a Hertz model at small compression ratios. The shear modulus was constant within the compression ratio range of 5-15%. Thus, the estimation of the shear modulus in this range was reliable. We found that the alginate beads had to be conserved in a calcium solution for one day so as to reach a stable state. The stability of the conserved beads was sustained for at least 2 w.

The thermal effect of the ultrasonic stimulation resulted in a linear decrease in the diameter of the beads with an increase in the solution temperature. The shear moduli of the beads showed an exponential increase with an increase in the solution temperature. In the absence of the ultrasonic thermal effect, no change in the shear moduli of the sonicated alginate beads was evident even when the sonication time was as high as 30 min. This can be attributed to the fact that alginate beads have a high shear modulus and that the ultrasonic cavitations cannot damage their surface. Even though the ultrasonic stimulation affected the physical properties of the gel matrix, the matrix recovered its initial state after the sonication. Because of their unique biological properties, alginate beads are frequently used as carriers in the biomedical and food industries. Thus, the practical application determines whether the cooling process should be used or not. In controlled release systems, ultrasonic stimulation can be used as a remote and noninvasive technique to control the release of the encapsulated substance. The association of alginate bead and ultrasonic stimulation will innovatively set up an externally controlled release system. In this work, a fundamental research on the effect of ultrasonic stimulation on the physical properties of alginate beads was carried out. The findings of this study are useful for practical applications.

Conflicts of interest

The authors declared that there is no conflict of interest.

Financial support and sponsorship

This work is supported and funded by the Department of Education, Henan Province (CN) (17A180036,17A180037), by National Natural Science Foundation of China (31600676) and by the International Science and Technology Cooperation Program of China (2015DFA30550).

References